| Main Page - Back |
|
From SudokuWiki.org, the puzzle solver's site |
Windoku Strategy
I'd like to thank and credit Mike Van Emmerik from Australia for this excellent idea he brought to my attention in March 2016. It makes a huge difference to understanding and solving Windoku puzzles.

Windoku is the same as Sudoku except it has four extra "boxes" we call "windows". Or does it? These extra boxes are additional constraints on the placement of numbers. 1-9 must fit uniquely in all four windows. But take a look at what Mike showed me in the first diagram.
Because these windows take up a lot of space, there doesn't seem much room down the sides and in the centre. In fact there are exactly nine cells in the three blue ringed groups in rows B, C and D. Because we have 1 to 9 twice over in the 'windows' 1 to 9 must also fit exactly in the remaining cells of those rows. This is as good as a window in its own right!
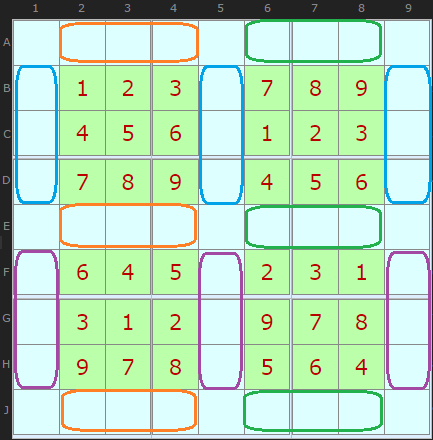
If that is true for those rows, and the puzzle is symmetrical, then it is true for rows F, G and H, and the columns. I've filled in all the extra windows from this logic.
This means you can consider them as disparate 'boxes' of 1 to 9 and use all the Sudoku strategies that rely in Sets and Locked Sets - which is pretty much all of them.
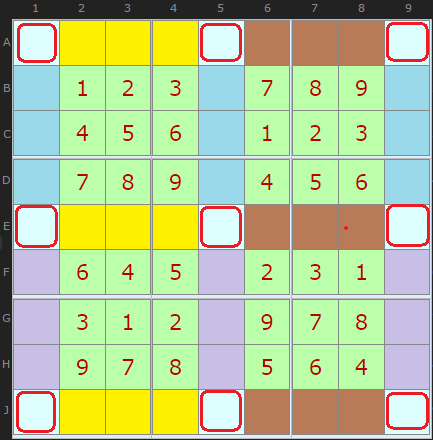
What's left? Magically, there are nine remaining cells, marked in red on the last diagram here. These cells belong to none of the windows so far but since there are exactly nine cells - they form the final 'window' shape. It mirrors the first set in the Colour Sudoku puzzle variant.
This is an elegant reduction of the puzzle and will give you many more opportunities to make eliminations. As of March 2016 the Windoku Sudoku takes full advantage of them.