XYZ-Wing
This is an extension of Y-Wing or (XY-Wing). John MacLeod defines one as "three cells that contain only 3 different numbers between them, but which fall outside the confines of one row/column/box, with one of the cells (the 'apex' or 'hinge') being able to see the other two; those other two having only one number in common; and the apex having all three numbers as candidates."
It follows therefore that one or other of the three cells must contain the common number; and hence any extraneous cell (there can only be two of them!) that "sees" all three cells of the Extended Trio cannot have that number as its true value.
It follows therefore that one or other of the three cells must contain the common number; and hence any extraneous cell (there can only be two of them!) that "sees" all three cells of the Extended Trio cannot have that number as its true value.
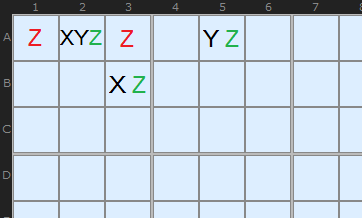
It gets its name from the three numbers X, Y and Z that are required in the hinge. The outer cells in the formation will be XZ and YZ, Z being the common number.
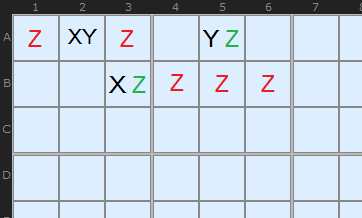
It is worth comparing the XYZ-Wing to the Y-Wing for a moment. Lets drop the Z candidate from the hinge. The diagram on the right is the result. With just two Zs in the pincer cells we get more cells elsewhere which could potentially contain a Z to eliminate. The overlap is greater with less cells to line up.
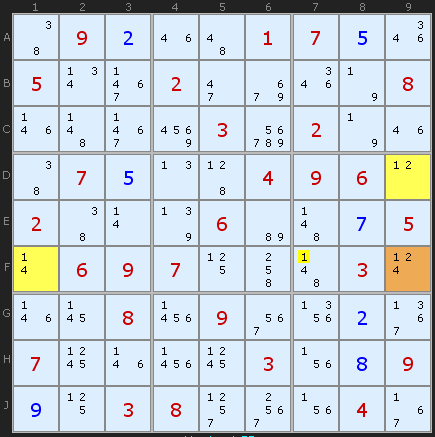
In this example the candidate number is 1 and F9 is the Hinge. It can see a 1/2 in D9 and a 1/4 in F1. We can reason this way: If D9 contains a 2 then F1 and F9 become a naked pair of 1/4 - and the naked pair rule applies. Same with F1. If that's a 4 then D9 and F9 become a naked pair of 1/2 each. If any of the three are 1 then 1 is still part of the formation. Any 1 visible to all three cells must be removed, in this case in F7.
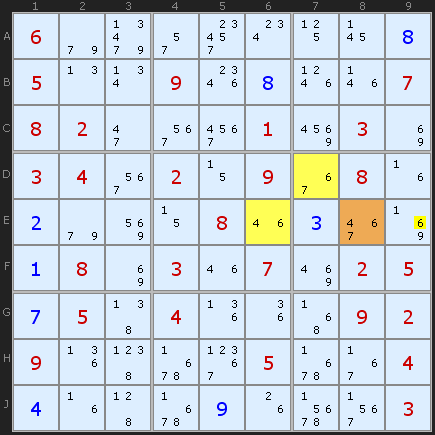
The hinge cell is on E8 and the common candidate is 6. The 6 in E9 can see all the 6s in the whole XYZ formation.
Aligned Pair Exclusion
The logic on an XYZ-Wing is completely different and lot simpler than the Aligned Pair Exclusion described below but the funny thing is that XYZ-Wing is a total sub-set of APE. Every XYZ-Wing can be solved by APE (but not vis versa).XYZ-Wing Exemplars
These puzzles require the XYZ-Wing strategy at some point but are otherwise trivial.They also require one Naked Pair.
They make good practice puzzles.

Comments
Email addresses are never displayed, but they are required to confirm your comments. When you enter your name and email address, you'll be sent a link to confirm your comment. Line breaks and paragraphs are automatically converted - no need to use <p> or <br> tags.
... by: Neil
Therefore, any cell containing a candidate 4 that can see both F1 and F9 can have that 4 removed.
Such a cell is F7.
There is still a 4 in F7 which could also be the answer on that row.
... by: Billy
The thing to remember with XYZ wings is that candidates have to see all 3 cells to be removed, unlike in Y-wings where a cell only has to see the wings.
... by: John
... by: JUN
THANKS
JUN
... by: scott
... by: Jon
... by: Pieter, Newtown, Australia
Beside Example 1 you say "the candidate number is 1 and F9 is the Hinge. It can see a 1/2 in D9 and a 1/4 in F1. We can reason this way: ... "
I find it interesting your reasoning or explanation here starts from the wings and then uses Naked Pairs, and doesn't work outwards from the Hinge (as you do in Y-Wings). I always seem to reason from the Hinge outwards. If F9 is a 2 then D9 is a 1, or if F9 is a 4 then F1 is a 1, or F9 could be a 1, therefore any 1 visible to all three cells must be removed, in this case in F7.
In WXYZ-Wing you explain it quite succinctly by saying "The easy principle is that each possible value of the hinge cell results in a Z value in one of the cells in the [WXYZ]-Wing pattern, thus leaving no room for a Z on any cell all [four] can 'see'."
I like the idea of "Bent Quads" for WXYZ-Wing, so I suppose XYZ-Wing could be considered a "Bent Triple"?!
... by: Richard Goodrich